Flowsnake or Gosper Curve

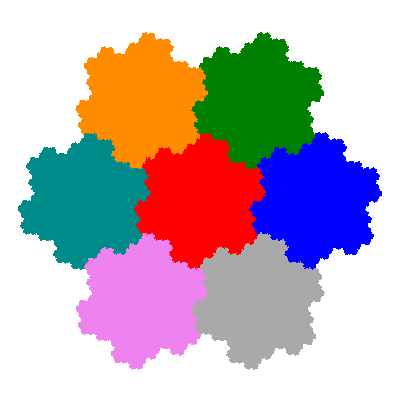
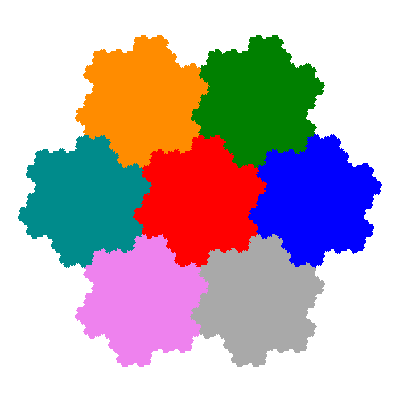
Because of symmetry there is only the one c6-symmetric cis-heptahextal, which however is 6⁷- (279,936-) fold degenerate, i.e. that are that number of distinct IFSs that generate the attractor. This figure was first introduced by William Gosper with a construction using a L-system.

If e_i,j denotes an Eisenstein integer then the IFS is

{ p → ap; p → ap + e_1,0; p → ap + e_1,1; p → ap + e_0,1; p → ap + e_-1,0; p → ap + e_-1,-1;p → ap + e_0,-1}

i.e. the vector elements of the transforms are 0 and the 6^th roots of 1.

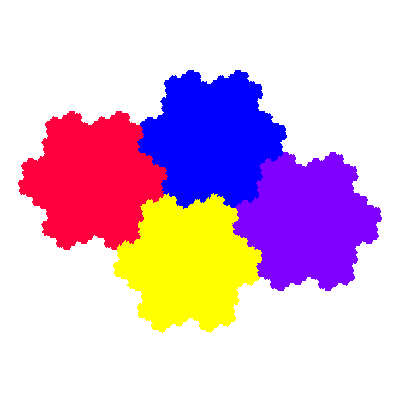
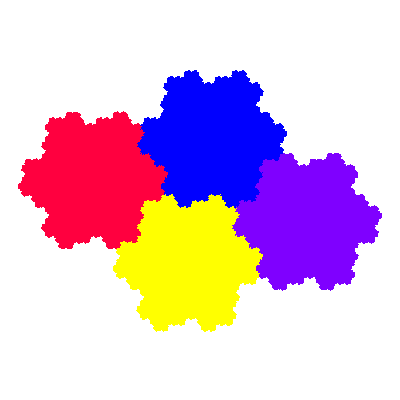
There is also an equivalent trans-heptahextal, with IFS

{ p → ap; p → ap + e_1,0; p → ap + e_1,1; p → ap + e_0,1; p → ap + e_-1,0; p → ap + e_-1,-1;p → ap + e_0,-1}

They both tile with plane with a single copy in the unit cell. For the IFSs given above the tiling vectors are e_3,1 and e_1,-2.

There are a great number of tiles derived from the cyclic heptahextals. This is a consequence of the breaking off symmetry in the derived tiles, which means that the high degeneracy of the tiles comes into play.

It turns out that symmetry reduces the number of distinct order 13 partial postcomposition derivatives to 7, 3 of which are not connected.

The number of order 7 grouped element tiles (in which the 6 elements of the outer ring are packed into fewer positions) is much larger, including (for each of the two tiles)

• teardrops: 6 elements packed into 1 position (2 tiles)
• pacmen: 6 elements packed into 5 positions (180 tiles)
• 6 elements packed into 4 consecutive positions
• 6 elements packed into 2 pairs of positions separated by single position gaps
• 6 elements packed into a triplet of positions and a single position separated by single position gaps
• 6 elements packed into 3 alternating positions
  • c3-propellors: 6 elements packed in pairs into 3 alternating positions (2 tiles)
  • 6 elements packed into 3 alternating positions but not wholly in pairs
• 6 elements packed into 3 consecutive positions
• 6 elements packed into 3 positions, neither wholly alternating nor wholly consecutive
• 6 elements packed into 2 opposite positions
  • c2-propellors: 6 elements packed in triplets into 2 opposite positions (2 tiles)
  • 6 elements packed into 2 opposite positions, but not in triplets
• 6 elements packed into 2 consecutive positions
• 6 elements packed into 2 positions, neither opposite nor consecutive

Grouped element tiles can also be derived from order 13 (dissection of the central elements) and order 19 (dissection of a pair of opposite elements) dissections of the basic tiles. The latter only has a c2-symmetric dissection, even though the attractor is c6-symmetric, so the number of distinct attractors is smaller - roughly 2¹⁹ versus 6¹³.

Source: Reversed engineered from William Gosper's L-system.

References:

© 2016 Stewart R. Hinsley