Some tiles associated with the 6th unit cubic Pisot number

Order 7 Paraallosymmetric Tiles (Overview)

Applying a composition of the partial postautocomposition and partial postallocomposition techniques to the order 3 symmetric tile produces 36 legitimate and fertile allosymmetric tile IFSs. Four of these are degenerate versions of a symmetric attractor, and would potentially give rise to 56 demisymmetric tiles. However they are disconnected, and disconnected in such a manner than it can be seen by inspection that all the candidate demisymmetric tiles are also disconnected. Sets of order 7 co-cell attractors include 4 “base” attractors and 124 “para” attractors with half the area of the “base” attractors. 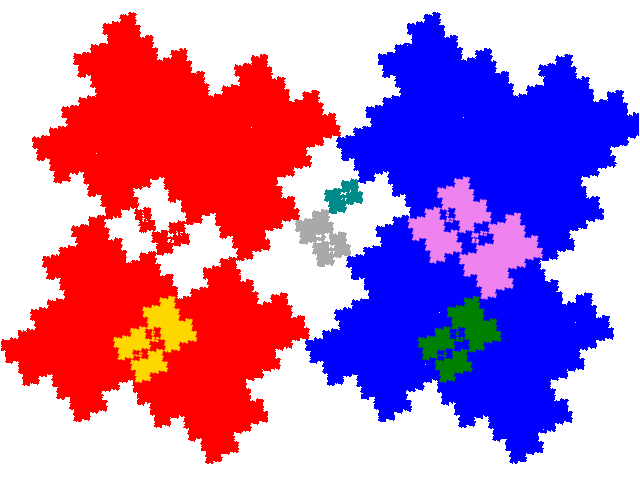Thus the 32 remaining IFSs can be divided among 8 sets of co-cell attractors, but these sets come in pairs differing by rotation by 180° around the origin, so only 4 sets have to be considered. There are two sets with dissection equation c+c²+3c³+c⁴+c⁵, one set with dissection equation c+2c²+c³+2c⁵+c⁷, and one set with dissection equation 2c+c⁴+2c⁵+c⁶+c⁷. Although all members of a set have a similarity dimension of 2, not all are disconnected, so the number of tiles in each set is a question requiring empirical investigation. They are 88, 73, 88 and 60 tiles in the sets respectively.

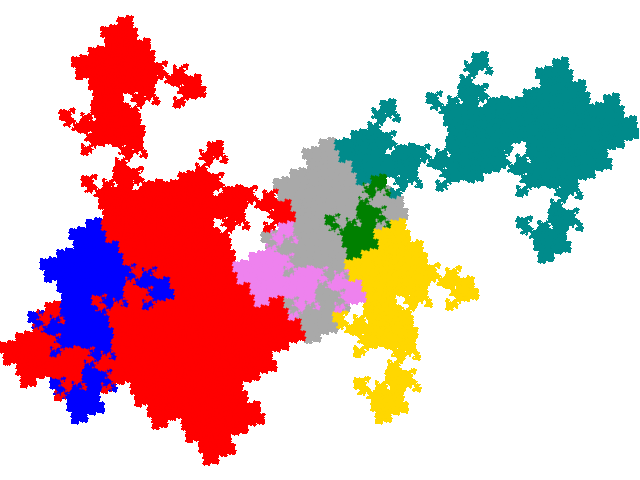	For any order 5 tile there are 32 attractors, including the original one. The same set of attractors is generated regardless of which asymmetric tile is started with. 8 of the attractors generate 6 partial postcomposition derivatives of the symmetric and demisymmetric tiles, and one is disconnected, leaving 88 novel tiles. simple teragons (8 tiles) some compact complex teragons (6 tiles) some more compact complex teragons (7 tiles) tiles with roughly triangular outlines (7 tiles) tiles with roughly triangular outlines (and bent corners) (7 tiles) tiles with large areas of 50% coverage (6 tiles) It turns out that all of these 23 tiles are fractional symmetric tiles, where 6 copies, made up of two copies each of three different sizes make up a symmetric tile. The cell transforms are `p→p` `p→-p` `p→ap - 1` `p→-(ap - 1)` The unit cells are shown on the pages for the various groups of tiles.

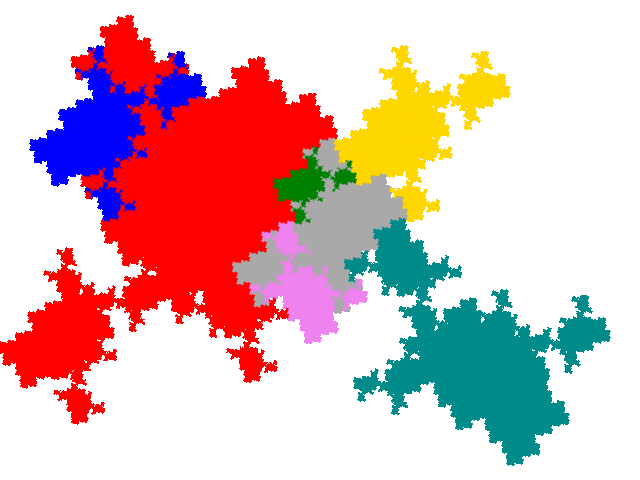
	The four tiles with dissection equation `c+2c²+c³+2c⁵+c⁷` all generate the same set of attractors. 36 of the attractors are disconnected, so this gives us 88 new tiles. These tiles all have 6 copies of the tile in the unit cell, with signature 001144.
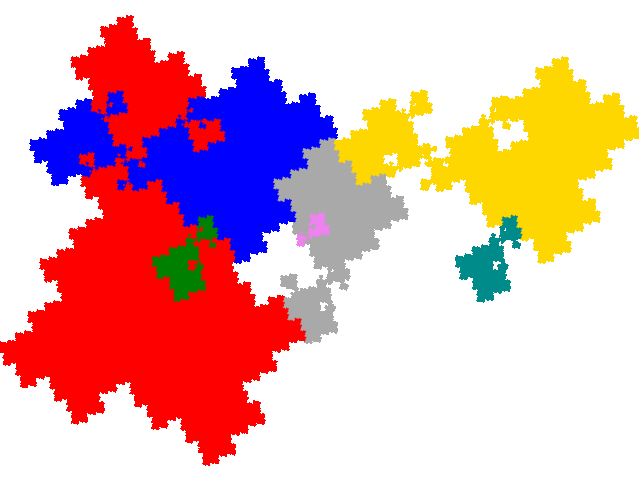
	The four tiles with dissection equation `2c+c⁴+2c⁵+c⁶+c⁷` all generate the same set of attractors. 64 of the attractors are disconnected, so this gives us 60 new tiles. The tiles can be divided into 4 groups differing in the size of the largest disclike part of the unit cell occupied solely by one copy of the tile. 1st group (4 tiles) 2nd group (8 tiles) 3rd group (32 tiles) 4th group (16 tiles) These tiles all have 6 copies of the tile in the unit cell, with signature 003344.